3D Shaping Studies
Detached from material specifications and a destined utility value, the focus on this project was to create a sphere knot that can be infinitely parqueted in xyz direction. Further more to create variation of this knot with transitions that feature different types of curvature shaping.
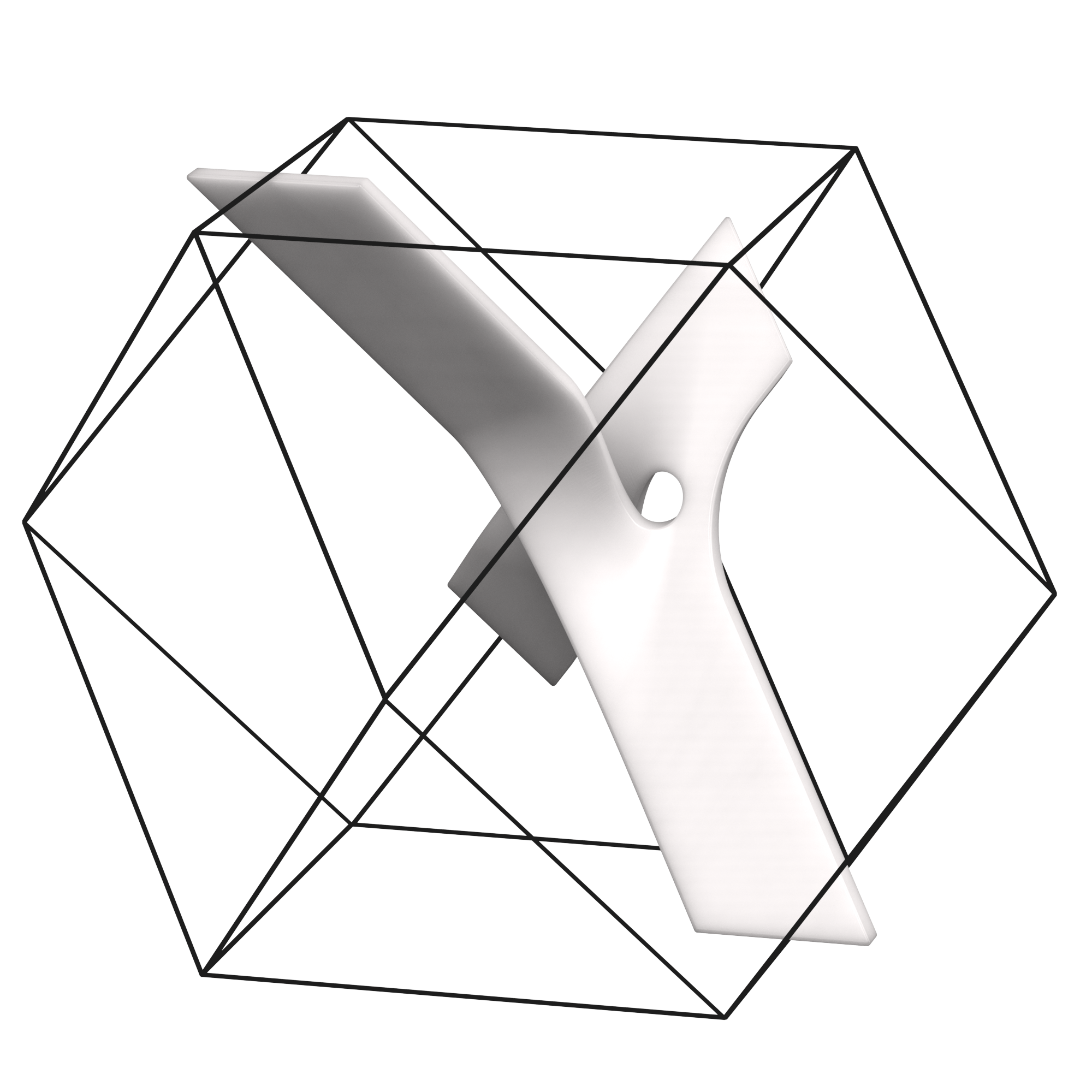
The first step is to define mathematical sphere solid, in this case a cuboctahedron. Second is to draw lines from outside edges that subtend in an intersection and define a sphere knot. The figure should be able to be parquetted within the main solid.
transitions Varieties
-

V1
The first variety comes up with concave & convex curvatures and slightly anticlastic transitions.
The treads are vertical splitted.
-

V2
The Treads in this figure are splitted lenghtways. Slightly distorted concave transitions conflate the splitted treads.
-

V3
The treads in the third variety conflate into a common circular cross section. The converging curvatures are distorted concave and synclastic.
Parquetting
-
The Modul can be placed four times within the cuboctahedron. The cuboctahedron is able to be parquetted in xyz direction infinitely and so is the fourpack of the sphereknot.

Utilization context
-
A immaginable transfer in a product context could be applicable in exerior architecture, e.g.
as a privacy shield.